選択した画像 if f(2tanx/1 tan^2x)=(1 cos2x)(sec^2x 2tanx)/2 then f(4)= 732420

 Ex 33, 23 Prove that tan⁡4𝑥 = (4 tan⁡〖𝑥 (1−tan2𝑥)〗)/(1 − 6 tan2 𝑥tan4 𝑥) Taking LHS tan 4x We know that tan 2x = (2 𝑡𝑎𝑛⁡𝑥)/(1 − 𝑡𝑎𝑛2 𝑥) Replacing x with 2x tan (2 × 2x) = (2 𝑡𝑎𝑛⁡2𝑥)/(1 − 𝑡𝑎𝑛2 2𝑥) tan 4x = (2 𝑡𝑎𝑛⁡2𝑥)/(1 − 𝑡𝑎𝑛2 2𝑥) = (2 taQuestion Prove The Identity Sec^2/2 Tan X = Csc 2x This problem has been solved! the opposite side is $$2tanx=2*2=4$$ the adjacent side is $$1tan^2x=12^2=14=3$$ (the negative sign just means it will not be in the first quad ) Using pythagoras the hypotenuse will be

What Is The General Solution Of Sec 2 2x 1 Tan 2x Quora

If f(2tanx/1 tan^2x)=(1 cos2x)(sec^2x 2tanx)/2 then f(4)=

If f(2tanx/1 tan^2x)=(1 cos2x)(sec^2x 2tanx)/2 then f(4)=-Prove as an identity;I am unable to see why $$1 \tan^2 x= 1/\cos^2x$$ I have looked into the topic anad I am familiar with the reciprocal ratios of cosec, sec, and cot but cannot derive how this statement makes sense Any help on the topic would be very much appreciated

2

∫ d x cos 2 ⁡ x = tan ⁡ x C 2 and by combining the two constants of integration into one, we find the answer (1) ∫ ( 1 tan ⁡ x) 2 d x = tan ⁡ x − 2 log ⁡ cosTan2x1 = sec2x sin 2x = 2 sin x cos x cos 2x = 2 cos2x 1 tan x = sin x cos x sec x = 1 cos x cot x = cos x sin x csc x = 1 sin x Some integration formulas R xn dx = xn1 n1 C R 1 x dx = lnjxjC R ex dx = ex C R sin x dx = cos x C R cos x dx = sin xC R if sinx=7/5 and angle x is in quadrant 2 and cos y=12/13 and angle y is in quadrant 1 find sin (xy) asked in TRIGONOMETRY by harvy0496 Apprentice doubleangle

Answer by Alan3354() ( Show Source ) You can put this solution on YOUR website! Ex 34, 8 Important Deleted for CBSE Board 22 ExamsYou are here Ex 34, 9 Important Deleted for CBSE Board 22 Exams Examples → FacebookWhatsapp Transcript Ex 34, 8Find the general solution of the equation sec2 2x = 1 – tan 2x sec2 2x = 1 – tan 2x 1 tan2 2x = 1 – tan2x tan2 2x tan2x = 1 – 1 tan2 2x tan2x = 0tan 2x (tan2x 1) = 0HenceWe know that sec2 x = 1I need to use the fact that $\tan 2x=\sin2x \ /\cos2x$ to prove that $$\tan 2x=\frac{2\tan x}{1\tan^2x}$$ I don't know where to start Please help or hint Thanks in advance

Click here👆to get an answer to your question ️ If sin x = cos^2x , then cos^2x (1 cos^2x) is equal to Join / Login > 11th > Maths Prove 4 sin 2 α cos 2 α 1 − 4 tan 2 α (1 Evaluate tan 2 θ − sec 2Trigonometry Solve for x tan (2x)= (2tan (x))/ (1tan (x)^2) tan(2x) = 2tan(x) 1−tan2 (x) tan ( 2 x) = 2 tan ( x) 1 tan 2 ( x) Since x x is on the right side of the equation, switch the sides so it is on the left side of the equation 2tan(x) 1− tan2(x) = tan(2x) 2 tan ( x) 1 tan 2 ( x) = tan ( 2 x)Get an answer for 'Prove the following sin 2x = (tan x)(1 cos 2x)' and find homework help for other Math questions at eNotes

Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco

Evaluate Lim X P 4 Sec 2x 2tanx 1 Cos4x Sarthaks Econnect Largest Online Education Community

 selected by subrita Best answer We have f (2tanx/ (1 tan2x)) = 1/2 (1 cos2x) (sec2x tanx) = 1/2x 2cos2x x (1 tan2x 2tanx) = cos2x x (1 tanx)2 = {cosx x (1 tanx)}2 = (cosx sinx)2For every tan 2x do I just use the formula sin theta / (1 cos theta)?Cos2 (x) (1 tan2 (x)) = 1 cos 2 ( x) ( 1 tan 2 ( x)) = 1 Replace the cos2(x) cos 2 ( x) with 1−sin2 (x) 1 sin 2 ( x) based on the sin2(x)cos2(x) = 1 sin 2 ( x) cos 2 ( x) = 1 identity 1−sin2 (x)(1tan2(x)) = 1 1 sin 2 ( x) ( 1 tan 2 ( x)) = 1 Simplify each term Tap for more steps

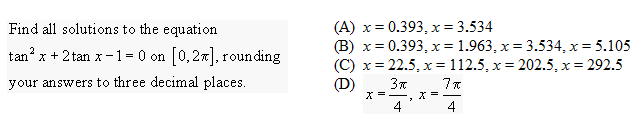
Solved Find All Solutions To The Equation Tan 2x 2tanx 1 0 Chegg Com

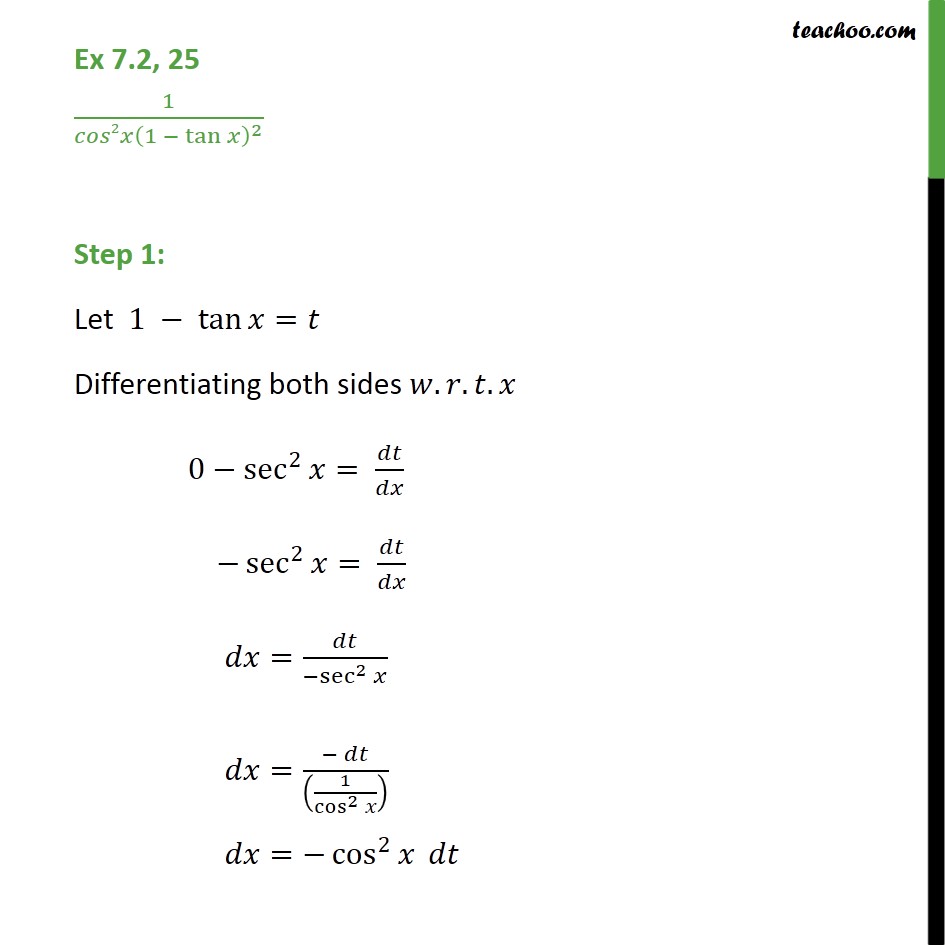
Ex 7 2 25 Integrate 1 Cos 2 X 1 Tan X 2 Ex 7 2

Let fk(x) = 1/k(sin^k x cos^k x) where x ∈ R and k ≥ 1 If 0 ≤ x the number of real values of x The sum of the radii of inscribed and circumscribed circles In a ∆PQR, if 3 sin P 4 cos Q = 6 and 4 sin Q 3 cos P = 1 If A = sin^2 x cos^4 x, then for all real xQuestion Decide whether the equation is a trigonometric identiye explain your reasoning cos^2x(1tan^2x)=1 secxtanx(1sin^2x)=sinx cos^2(2x)sin^2=0Find sin (2x), cos (2x), and tan (2x) from the given information tan (x) = 1/2, x in quadrant I *** Hypotenuse of reference right triangle in quadrant I=√(1^22^2)=√5

Solved Question 1 Integrate The Following Function Sec 2x Chegg Com

いろいろ Tan2x 1sec2x ただの悪魔の画像

You can put this solution on YOUR website! 1 tan^2 x = 3 tanx =±√3 , so x is in all 4 quadrants x = 60° , 1° , 240° , 300° 2 tanx sin^2 x = tanx tanx(sin^2 x 1) = 0 tanx = 0 or sinx = ± 1Verify that $$ 2\cos^2x1 = \frac{1\tan^2x}{1\tan^2x}$$ Stack Exchange Network Stack Exchange network consists of 178 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers

いろいろ Tan2x 1sec2x ただの悪魔の画像

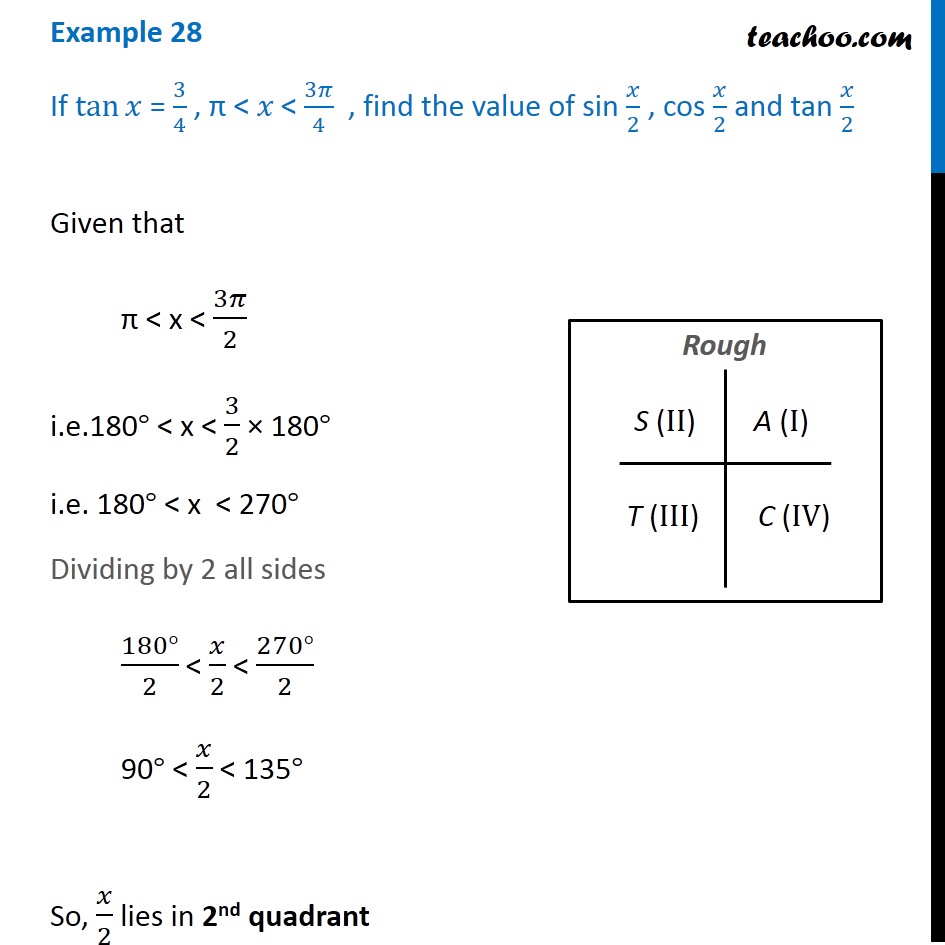
Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2

RHs = (2 tan x cos 2 x 1 ) cos 2 x = 2 sin x cos x 1 = 1 sin 2 x If sin 2 x = t, then we have f t = 1 t, where t = sin 2 x where − 1 ≤ t ≤ 1 ∴ Domain is − 1, 1 Adding 1 throughout, 0 ≤ 1 t ≤ 2 or 0 ≤ f (t) ≤ 2 ∴ Range of f(t) is 0, 2Chapter 4 Taylor Series 17 same derivative at that point a and also the same second derivative there We do both at once and define the second degree Taylor Polynomial for f (x) near the point x = a f (x) ≈ P 2(x) = f (a) f (a)(x −a) f (a) 2 (x −a)2 Check that P 2(x) has the same first and second derivative that f (x) does at the point x = a 43 Higher Order Taylor PolynomialsSin(2x) = (2tan(x)) / (1tan^2(x)) *** Start with RHS 2tanx/(1tan^2x) 2tanx/(sec^2x) 2(sinx/cosx)/(1

Calculus I Ii Amp Iii For Mahavier S Class Math Nerds

2

 Tan^2 x = 1 cos2x/ 1 cos 2x andrianartic9331 andrianartic9331 Mathematics College answered • expert verified True or false Tan^2 x = 1 cos2x/ 1 cos 2x 2 See answers AdvertisementRewrite sec(x) sec ( x) in terms of sines and cosines Rewrite tan(x) tan ( x) in terms of sines and cosines Multiply by the reciprocal of the fraction to divide by 1 cos(x) 1 cos ( x) Write cos(x) cos ( x) as a fraction with denominator 1 1 Cancel the common factor of cos(x) cos ( x) Use the identities $1 tan^2(x)=sec^2(x)$, $1cot^2(x)=cosec^2(x)$ and the definitions of the reciprocal trig functions This will give the answers up to an unknown sign, for which we need to known whether x is obtuse or acute

Qprove That Tan X 2 Tan 2x 4 Tan 4x 8 Cot 8x Cot X Mathematics Topperlearning Com Blpusg55

いろいろ Tan2x 1sec2x ただの悪魔の画像

Simplify each term Tap for more steps Multiply cos ( x) cos ( x) by 1 1 cos ( x) tan 2 ( x) cos ( x) tan 2 ( x) Rewrite tan ( x) tan ( x) in terms of sines and cosines cos ( x) ( sin ( x) cos ( x)) 2 cos ( x) ( sin ( x) cos ( x)) 2 Apply the product rule to sin ( x) cos ( x) sin ( x) cos ( x) cos ( x) sin 2 ( x) cos 2 ( x) cosThe first derivative of the trigonometric function of tangent with respect to its argument is the reciprocal of the trigonometric function of cosine squared Snarf! f(2tanx/1tan 2 x)=(cos2x1) (sec 2 x2tanx)/2 then f(4) is equal to?

2

If F 2tanx 1 Tan2x Then F 4 Youtube

 sec=4 find sin(2x), cos(2x), tan(2x) These problems seem straight forward but I keep getting the wrong answer If you could please in detail show me how to solve this I would really, really, really appreciate it so I don't throw my Trig book out the windowSee the answer Show transcribed image text Expert Answer 100% (3 ratings) Previous question Next question Transcribed Image Text from this Question Prove the identity sec^2/2 tan x = csc 2xWeekly Subscription $249 USD per week until cancelled Monthly Subscription $799 USD per month until cancelled Annual Subscription $3499 USD per year until cancelled

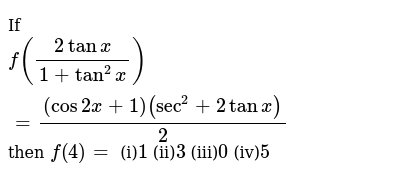
If F 2tanx 1 Tan 2 X Cos2x 1 Sec 2 2tanx 2 Then F

Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube

Answer (1 of 10) \int \frac{1\tan^2x}{1\tan^2x} \,dx \int \frac{1\tan^2x}{\sec^2x} \,dx \int \frac{1\tan^2x}{\frac{1}{\cos^2x}} \,dx \int \cos^2x(1\tan^2x) \,dxAnswer (1 of 3) ( 1 tanx/2) / (1 tanx/2) =( cosx/2 sinx/2)/(cosx/2 sinx/2) Multiplying numerator and denominator by the denominator we get (cos^2 x/2 sin^2(tan^2(x)1)/sec^2 = 12cos^2(x) Mulitiply by cos^2 (sin^2 cos^2)/1 = 1 2cos^2 Add cos^2 sin^2 = 1 cos^2 QED Answer by MathTherapy(91) (Show Source) tan^4 (x) 1 or (tan^2(x)1)(tan^2x1) then i'm stuck!

Solved Find All Solutions To The Equation Tan 2x 2tanx 1 0 Chegg Com

Tan2x ただの悪魔の画像

  Use the fact that tanx = sinx cosx and sin2x = 2sinxcosx So 2 sinx cosx ⋅ 1 1 sinx cos2x = 2sinxcosx 2 sinx cosx ⋅ cos2x cos2x sin2x = 2sinxcosx 2 sinx cosx ⋅ cos2 x cos2x sin2x = 2sinxcosxIf $6\tan^2 x3\cos^2x=\cos 2x$, then what is the value of $\cos 2x$?You can put this solution on YOUR website!

F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2

Solved Prove Or Disprove The Given Identity Sec 2 X Chegg Com

Simply tan (AB) = tanAtanB/1tanAtanB From this formula we can derive tan (2x) as tan (xx) So tan (2x)= 2tanx/1tanxtanx We can always go forCan anyone give me some hints for this?Introduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formula

Derivatives Of Inverse Functions From Equation Video Khan Academy

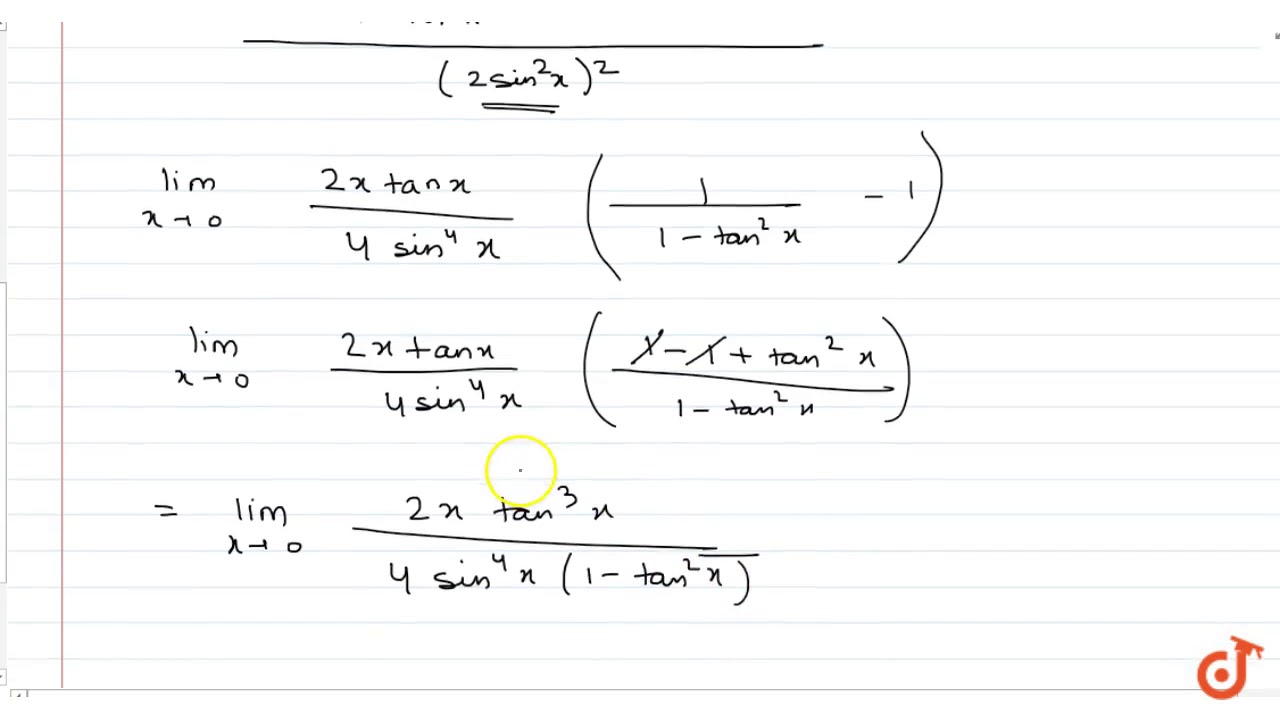
The Value Of Lim X Gt0 Xtan2x 2xtanx 1 Cos2x 2 Is Equal To Youtube

Weekly Subscription $249 USD per week until cancelled Monthly Subscription $799 USD per month until cancelled Annual Subscription $3499 USD per year until cancelledAnswer (1 of 7) Here, tan x = 1/2 We know that, the relation between tan x and sec x is sec^2xtan^2x=1 Using this relation, first, we will determine the value of cos x and then, we will determine the value of sin x So now, sec^2xtan^2x=1 => sec^2x(1/4)=1 => sec^2x=1(1/4) => sec^2x= Get an answer for 'verify (1 tan^2x)/(tan^2x) = csc^2x' and find homework help for other Math questions at eNotes

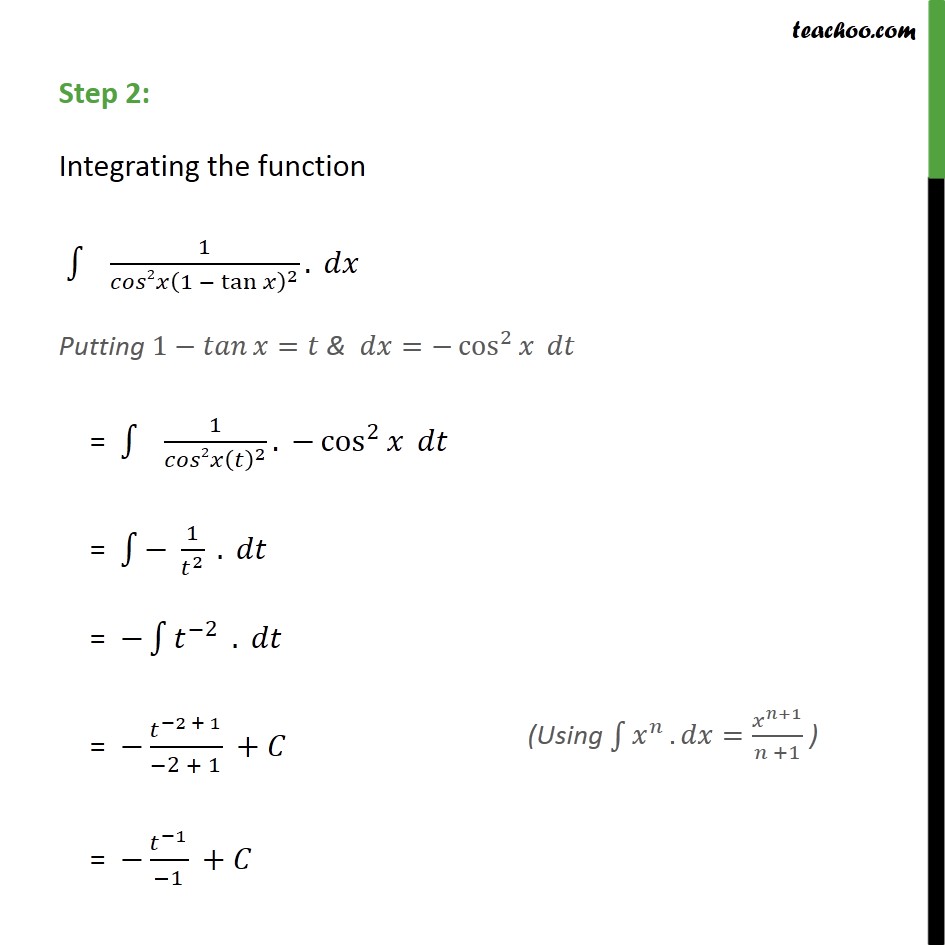
Ex 7 2 25 Integrate 1 Cos 2 X 1 Tan X 2 Ex 7 2

2

Solve for x sec(x)^22tan(x)=4 Replace the with based on the identity Reorder the polynomial Factor using the AC method Tap for more steps Consider the form Find a pair of integers whose product is and whose sum is In this case, whose product is and whose sum isShare with your friends Share 0TRIGONOMETRIC EQUATIONS An equation involving one or more trigonometrical ratios of unknown angle is called a trigonometric equation eg cos 2 x – 4 sin x = 1 It is to be noted that a trigonometrical identity is satisfied for every value of the unknown angle where as trigonometric equation is satisfied only for some values (finite or infinite) of unknown angle

How Do You Solve Sec 2x 2tanx 0 Socratic

Tan 2x Formula What Is Tan 2x Formula Examples

B) (tanx 1)(tanx1)/1 tan^2(x) = (sinx/cosx 1)(sinx/cosx 1) / 1 Get an answer for 'Show that `tan^2 x = (1 cos(2x))/(1 cos(2x))`' and find homework help for other Math questions at eNotes Search this site Go iconquestion Tyrion101 said But is it equal to (2tanx/1tan^2x)^2 is what I'm asking I may have been unclear Yes and no means , which in turn is equal to In what you wrote, you are missing parentheses around the quantity in the denominator, 1 tan 2 (x) What you wrote is the same as #10 symbolipoint

Is Tanx 2 2tanx 1 5tanx Sec X Quora

Old Version Trig Show Sin 2x 2 Sin X Cos X 2tan X 1 Tan 2 X Youtube

 Example 5 Express tan−1 cos⁡x/(1 − sin⁡x ) , – π/2 < x < 3π/2 in the simplest form Lets first calculate cos x & 1 – sin x We know that cos 2x = 𝐜𝐨𝐬𝟐⁡𝐱 – 𝐬𝐢𝐧𝟐⁡𝐱 Replacing x by 𝑥/2 cos (2x/2) = cos2 x/2 – sin2 x/2 cos x = cos2 x/2 – sin2 x/2 We know that sin 2x = 2 sin x

Cos 2x

Solved Find All Solutions To The Equation Tan 2x 2tanx 1 0 Chegg Com

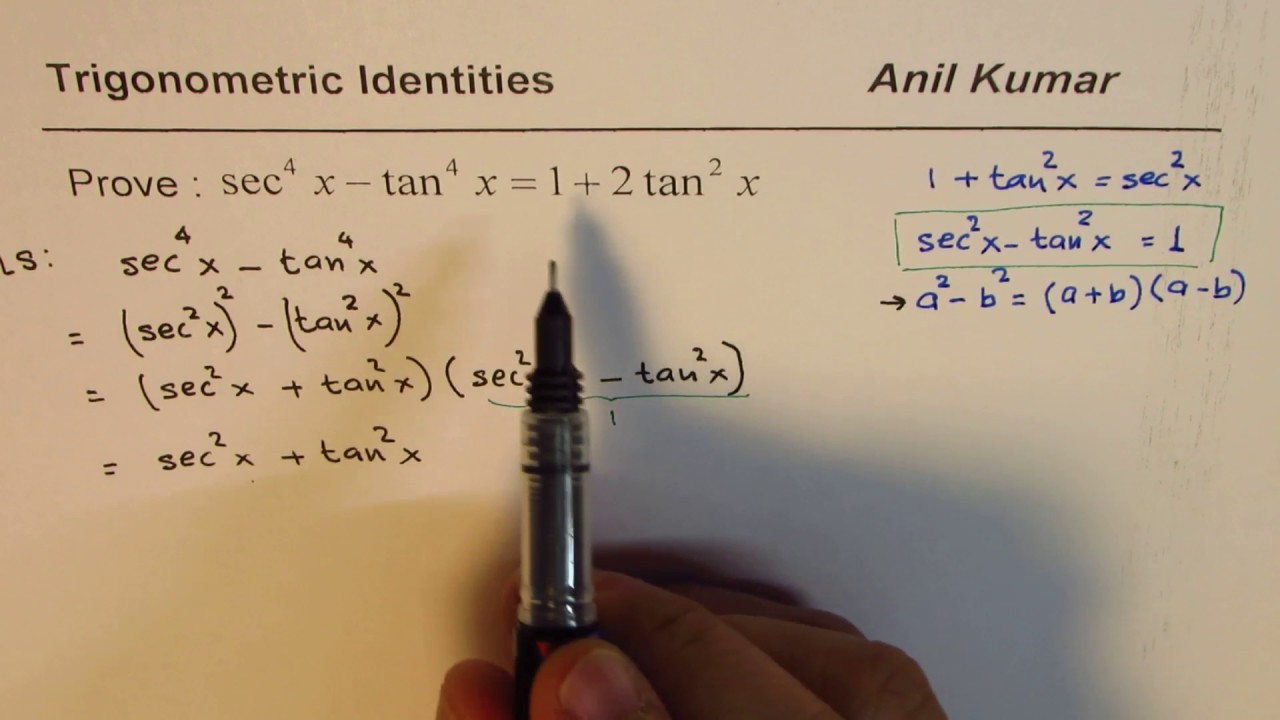
Trig Identity Sec 4x Tan 4x 1 2tan 2x Youtube

Which Of The Following Are Solutions To 2tanx 1 Tan 2x Sqrt 3 Check All That Apply Options Are In Brainly Com

How To Find Least Value Of Cos 2 X Sec 2 X Quora

Tanx 4 3 X In Quadrant Ii Find The Value Of Sinx 2 Cosx 2 Tanx 2

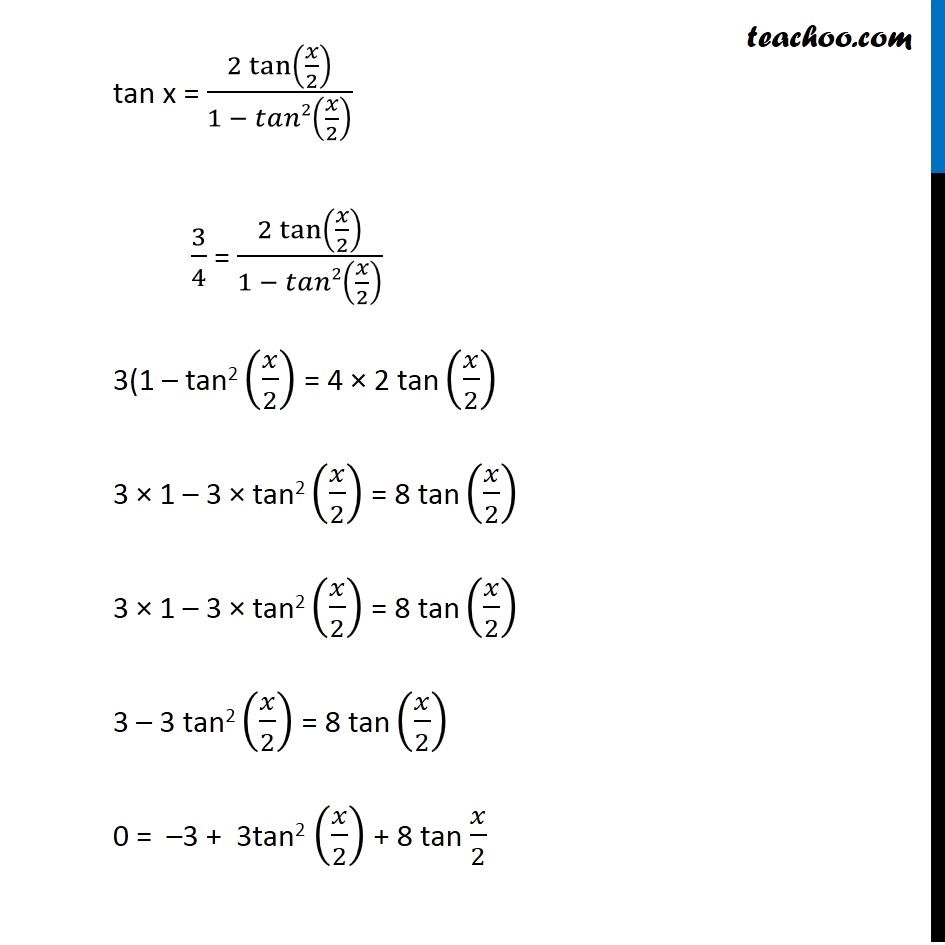
Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

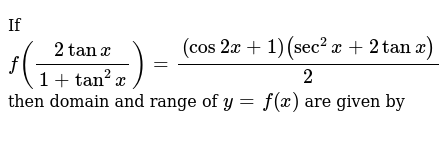
いろいろ Tan2x 1sec2x ただの悪魔の画像

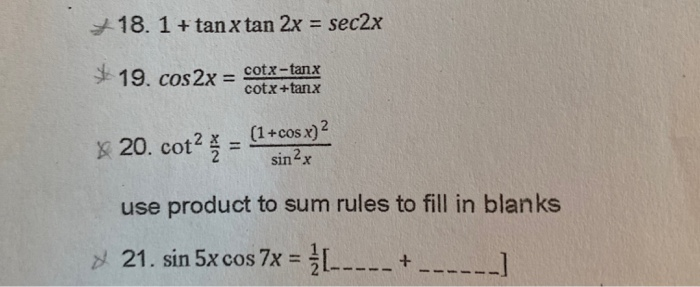
Solved 18 1 Tan X Tan 2x Sec2x 19 Cos2x Cotx Tank Chegg Com

2

Trigonometric Identity With Pythagorens Sec 2x Sin 2x Cos 2x Tan 2x Youtube

Tan2x 1 ただの悪魔の画像

Tan2x 1 ただの悪魔の画像

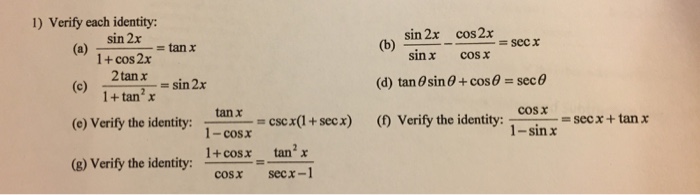
Solved Verify Each Identity A Sin 2x 1 Cos 2x Tan X Chegg Com

Derivatives Of Tan X And Cot X Video Khan Academy

いろいろ Tan2x 1sec2x ただの悪魔の画像

2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula

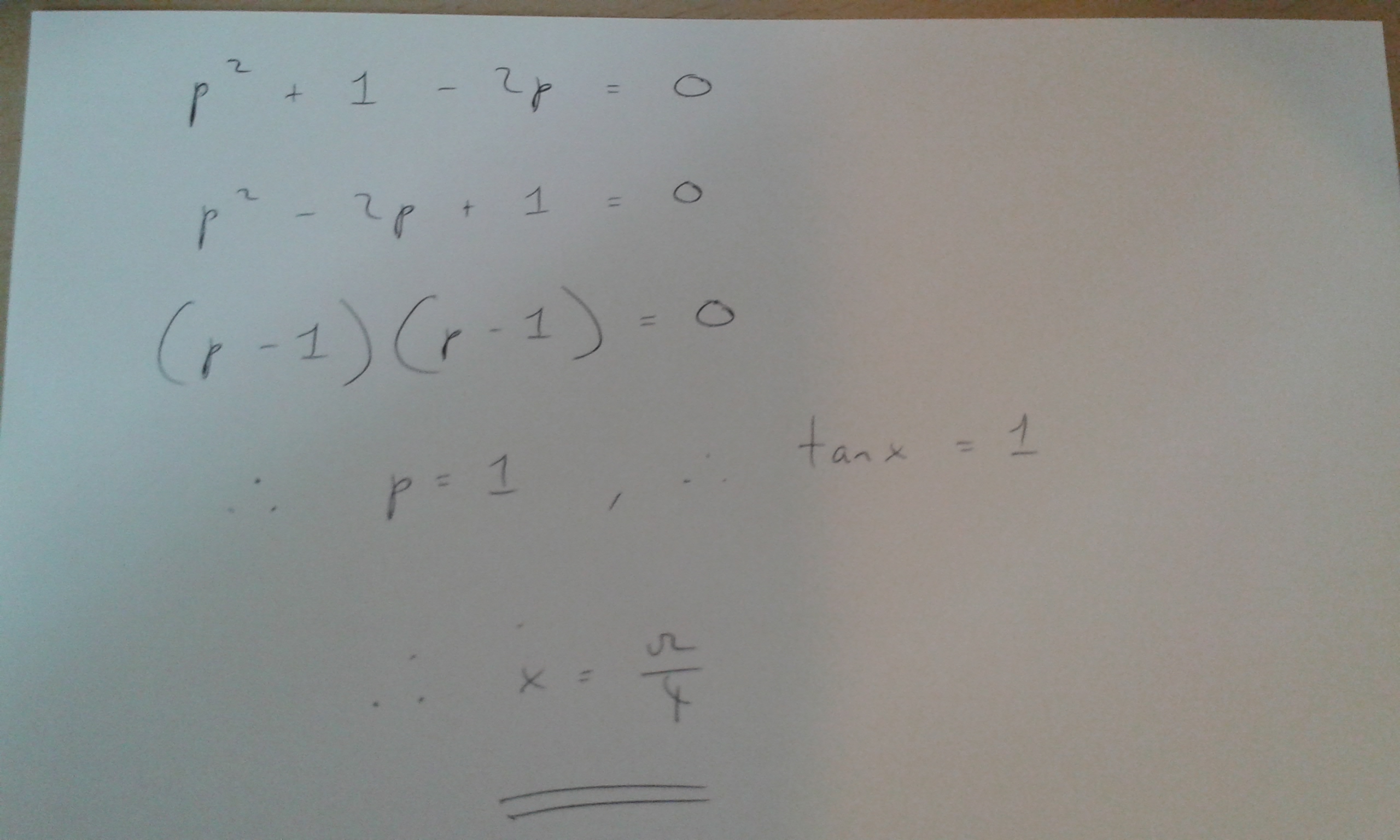
How Do You Solve Sec 2x 2tanx 0 Socratic

いろいろ Tan2x 1sec2x ただの悪魔の画像

Is Tanx 2 2tanx 1 5tanx Sec X Quora

Tan2x ただの悪魔の画像

1 Tan 2x 1 Tan 2 X 1 2cos 2 X Youtube

Derivative Rules For Y Cos X And Y Tan X Calculus Socratic

Limit X 0 Xtan2x 2xtanx 1 Cos2x 2 Equals

How To Prove 2 1 Cosx Tan 2 X 2 1 Quora

A 1 Tan X Tan X 1 Show That A Ta 1 Cos 2x Sin 2x Sin 2x Cos 2x Sarthaks Econnect Largest Online Education Community

Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo

What Is The Tangent Line For The Function F X Cos 2x At X Pi 6 Quora

If Cos 2x Cos 4x 1 Then Tan 2x Tan 4x 1 1 2 0 3 1 4 2

1

5 If F 2tan X 1 Tan 2 X Qquad Cos2x 1 Sec 2 X 2tan X

Integral Of 1 Cos 2 X Sqrt 1 Tan X Calculus 1 Trig Integrals Youtube

Integral Of Sec 2 X Sqrt 1 Tan 2 X Using The Arcsine Function Youtube

How Do You Solve 1 Tan 2x 6 2sec 2x Socratic

1 Tan 2x Sec 2x

If F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2 Then F 4

1 Tan 2x 1 Cos 2x Sin 2x 2sin 4x 1 Sin 2x Trigonometric Identities Mcr3u Youtube

Tan2x ただの悪魔の画像

2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula

Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2

If Tan X Sec X 4 Then What Is The Value Of Sin X Quora

If X 30 Verify That I Tan2x 2tanx 1 Tan 2x Ii Sinx Sqrt 1 Cos2x 2

Solved Prove The Identity Cot 2x 1 Tan2 X 2 Tan X Chegg Com

What Is The Integration Of Tan 2x Solution Quora

If F 2tanx 1 Tan 2x Cos2x 1 Sec 2x Tanx 2 Then F X

Sec 6x Tan 6x 1 2 Tan 2x Sec 2x Important Difficult Trigonometric Identity Youtube

Pw7jvk9kgjfojm

How To Prove Math Dfrac Sqrt 1 Cos 2x 1 Cos 2x Tan X Math Quora

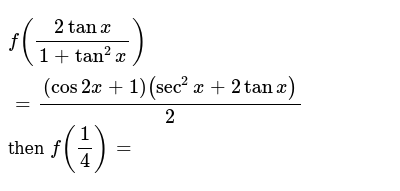
F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2 Then F 1

What Is The General Solution Of Sec 2 2x 1 Tan 2x Quora

Tan2x ただの悪魔の画像

Exponential Functions Frac D D X Left X E X Right Y Y X E 2

Prove That 1 Sin 2x Cos 2x 1 Sin 2x Cos 2x Tan X Youtube

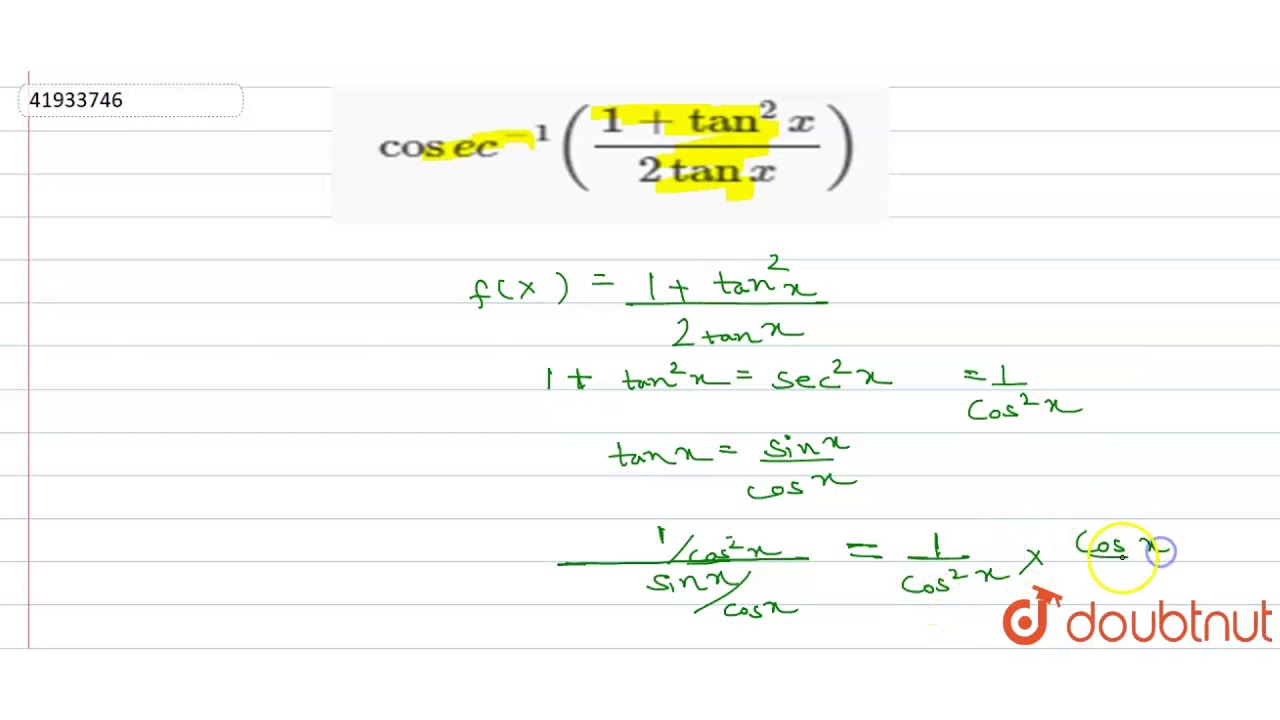
Cosec 1 1 Tan 2x 2tanx Youtube

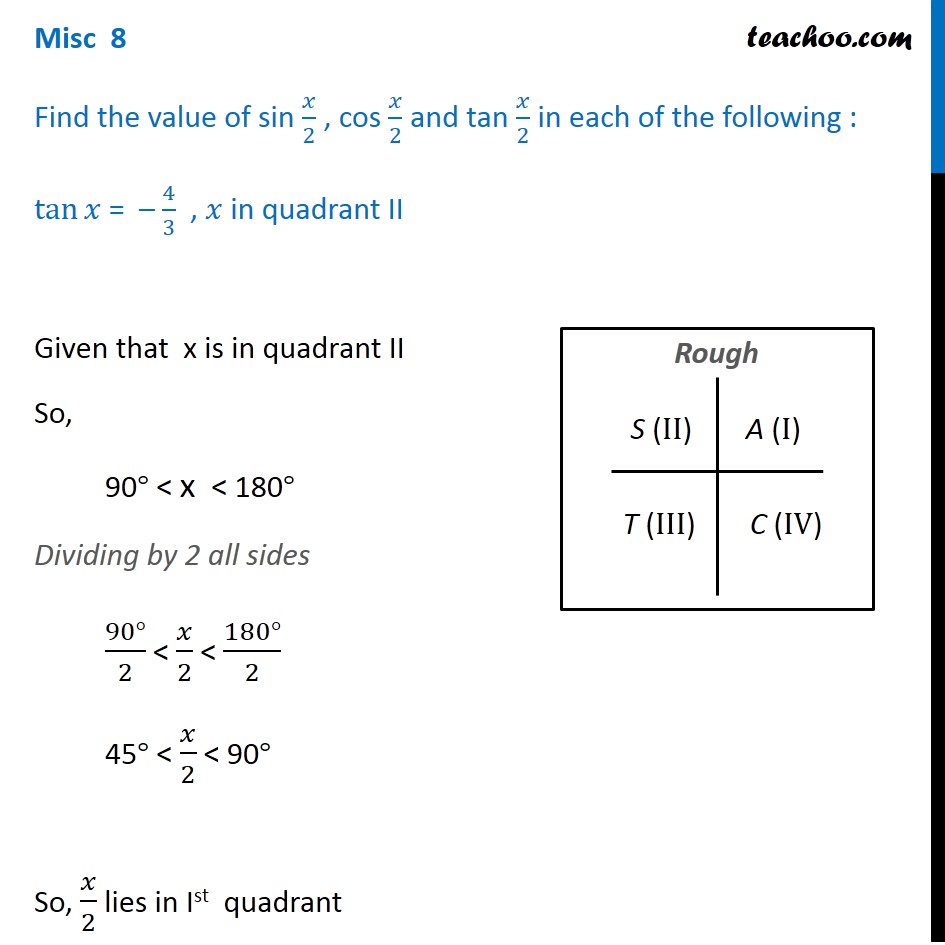
Misc 8 Tan X 4 3 Find Sin X 2 Cos X 2 And Tan X 2

Relating Trigonometric Functions Trigonometry Socratic

What Is The Value Of Tan2x Tan4x 1 Find Tan3x Quora

2tanx 1 Tan 2x 6181 2tanx 1 Tan 2x Formula

2

Find The Range Of Functionfx 1 2tan 11 X2 P 4

1

Cos 2x

If F 2tanx 1 Tan2x Then F 4 Youtube

1

How To Find Least Value Of Cos 2 X Sec 2 X Quora

F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2

bestpictjcry Tan 2x Tan 2x

Tan2x ただの悪魔の画像

2

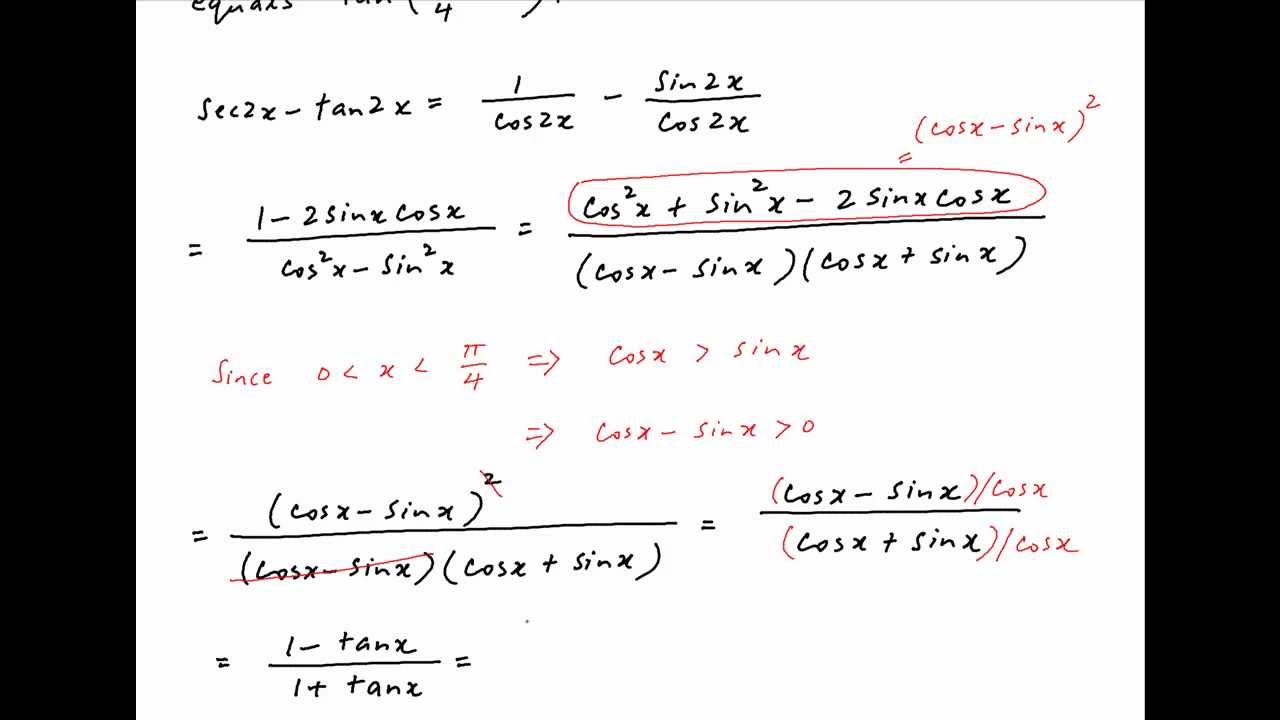
Iit 1994 Prove That Sec2x Tan2x Tan Pi 4 X When X Lies Between 0 And Pi 4 Youtube

Lim X Tan 2x 2x Tan X 1 Cos 2x 2 For X 0 Is Sarthaks Econnect Largest Online Education Community

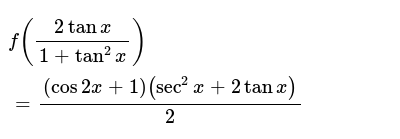
F 2tanx 1 Tan 2x Cos2x 1 Sec 2x 2tanx 2

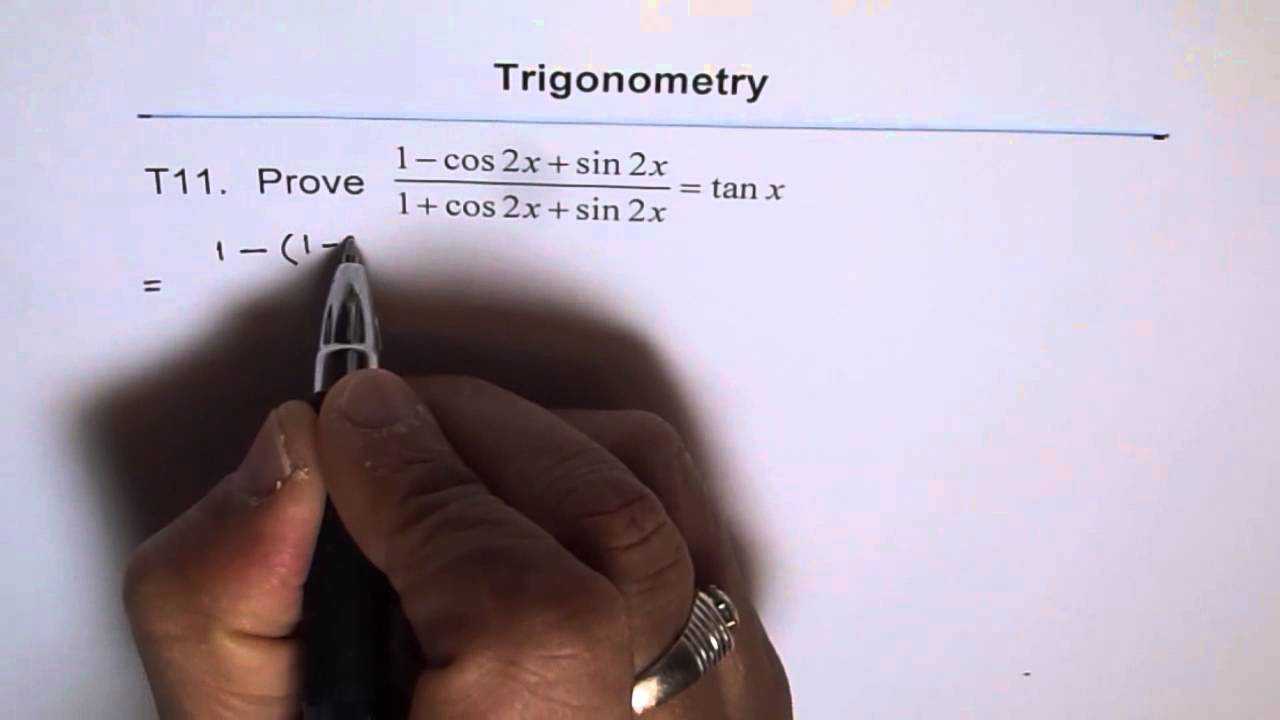
Trigonometric Identity 1 Cos2x Sin2x 1 Cos2x Sin2x Tanx Youtube

Domaine De Definition De Tan 2x

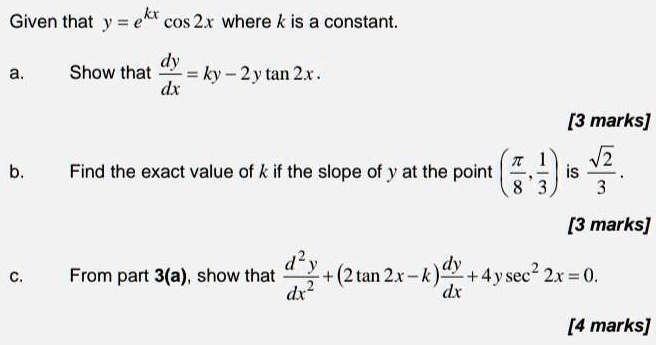
Nwoqnovhk C Gm

If F 2tanx 1 Tan 2x 1 Cos2x Sec 2x 2tanx 2 Then F 1 Brainly In